Van Hove singularities and nonadiabatic effects in superconductivity
Abstract
The breakdown of Migdal's theorem implies the generalization of the electronphonon theory of superconductivity to include various nonadiabatic effects. The reduced electronic screening due to the small carrier density and the presence of a van Hove singularity forthe optimized cuprates complete a new scenario for both the superconductive and the normalstate. In particular the van Hove singularity can be related to highest values of Tc, to thereduction of the isotope effect and to the linear resistivity.
A common feature of all unconventional superconductors: cuprates, fullerene compoundsand organics is the extremely small carrier density, one or two orders of magnitudes smaller than in usual metals. This situation has two fundamental consequences: on the one handelectrons (or holes) are strongly correlated and, on the other hand, it locates these systems outside the range of validity of Migdal's theorem. The small carrier density corresponds to a very unfavorable situation with respect to the usual BCS-Eliashberg theory becausethe average density of states (DOS) is rather small and the residual Coulomb repulsion is certainly larger than in usual superconductors. An additional element which is characteristic of the oxides is the presence of a van Hove singularity (vHs) in the DOS near the Fermi level. In our opinion, however, there is a hierarchy of importance between the three elements previously mentioned. Namely the breakdown of Migdal's theorem requires the generalization of the Eliashberg equations to the nonadiabatic regime. This is what we have done recently, and the main result is that, in a favorable situation of positive vertex corrections etc., the nonadiabatic effects can appreciably raise Tc and lead to a number of new effects for both the superconductive and normal state. This result is particularly appealing because it allows us to understand the positive role of the small carrier density in these systems. Within this frame work of nonadiabatic superconductivity the role of electronic correlations and the vHs is to locate the system in a favorable range of parameters. In this perspective the main effect of electronic correlations is to favor small momentum scattering, leading to positive nonadiabatic effects. As for the vHs the situation is more delicate because, apartfrom the value of the total bandwidth, the vHs intrinsically implies a breakdown of Migdal'stheorem and it can only be studied consistently by including the nonadiabatic effects.In this letter we focus in particular on the interplay between nonadiabatic effects and vHs.The effect of electronic correlations is parameterized by a modulation of the electron-phononcoupling as a function of momentum. We show that with these elements it is possibleto understand in a coherent way many properties of both the superconductive and normalstate. In particular the small carrier density can be related to an enhancement of Tc via thenonadiabatic effects. The vHs ampli¯es these effects and provides a further enhancement of Tcassociated to a decrease of the isotope effect. In addition, for the normal state, the above elements lead to a rather complex generalization of the usual Fermi liquid whose properties can reproduce many of the observed anomalies. The Migdal parameter is m = D=EF, where D is the Debye frequency and EF the Fermienergy. The usual Eliashberg theory of SC includes the self-energy effects to all orders in (dimensionless el-ph coupling) in the limit m = 0. The extension of Eliashberg equations tothe nonadiabatic regime includes the ¯rst-order effects with respect to the parameter and changes qualitatively the structure of the theory. In some sense this change is of thesame order as the change from BCS to Eliashberg, once one includes the self-energy effects. Inaddition this new theory preserves, in some sense, the Fermiliquid nature of the system butin a more complex phenomenology. Which seems actually to be the situation in high-Tc materials.An essential element in the nonadiabatic theory of superconductivity is the structure of the vertex correction function

expressed in usual Matsubara notations. The vertex function PV depends essentially on theexchanged frequency and the momentum q, and contains as parameters the phonon frequency 0 (single Einstein mode) and the bandwidth E = 2EF, considering thehalf-¯lled case. A detailed study of this function shows that it is positive in the dynamical limit (smallq and large), while it is negative in the static limit (large q and small). This leads tothe result that small q scattering is associated to positive vertex corrections and there for eto an enhancement of Tc. This result shows that the common opinion that vertex corrections are, in general, negative, is actually incorrect. The predominance of smallq scattering is a characteristic of strongly correlated electrons. In addition, the presenceof a vHs strongly enhances the phase space for small q scattering within the same vHs andtherefore leads to a further enhancement of Tc. In order to study the effect of a vHs on the vertex corrections, we consider the simpledispersion on the saddle point to which there corresponds a logarithmic, where E is the bandwidth and N0 is the average DOS over the wholeband. We consider the Fermi level just on the singularity and assume an isotropic dispersion,so that the vertex function will depend only on q = jqj. We also consider only the case n = 0in order to derive analytical expressions that have also been tested numerically.
In a normal situation vertex corrections are of order l x m. In the presence of a vHs we obtain instead

The nonadiabatic generalization of Eliashberg equations implies the inclusion of vertexcorrections and of the cross phonon diagram. We include the complex dependence ofvertex (PV) and cross (PC) diagrams on momentum and frequency by performing a weighted average on q and. In order to reproduce the effect of electronic correlations, we introduce anupper momentum cut qc in the structure of the el-ph interaction g(q). In addition thisfunction is normalized in such a way that the total coupling strength is constant for different qc. The generalized equations for the superconducting instability can be written as


The gap equation can be solved analytically by factorizing the gap kernel Dn-m = Dn x Dm and the final expression for the critical temperature in the nonadiabatic van Hove scenariois

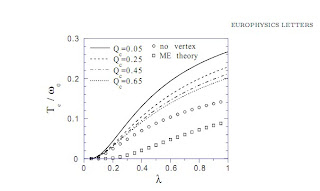
The behavior of Tc as a function of (l) is reported for a fixed value of m=0,1. It shows a remarkable increase of the critical temperature due to the vertex and cross terms with respect to the Migdal-Eliashberg adiabatic case, especially for small values of (l). Note that such an enhancement is evident also for relatively small values of m (m=0,1). Moreover, the ratio of the nonadiabatic vs. the adiabatic Tc would be increased even more by the introduction

Scheme of two scattering processes for the resistivity due to electron-phonon scattering.

Behavior of the resistivity as a function of temperature derived from Boltzmann theory and including both the normal and Umklapp scattering.
In our perspective, therefore, all the HTSC, i.e. oxides, fullerene and organics, wouldbe very unfavorable systems from the BCS-Eliashberg point of view, corresponding to nosuperconductivity at all, or with extremely small values of Tc<<1k. tc ="10-40K." tc="40K)">
ORLANING COLMENARES
ELECTRONICA DEL ESTADO SOLIDO
Visita mi BLOG:
BIBLIOGRAFIA:








No hay comentarios:
Publicar un comentario